
the 'range is found by subtracting the lowest value from the highest value.median), third and fourth quartile.ĭiscuss how some features of a display can be labelled directly, while other features are found by calculation: Similarly, annotate the quartiles and discuss the meaning of the first, second (i.e. Point to some outliers and ask what they could represent. Summarise the meaning of 'median' for students and annotate the median on the display. If students are not familiar with the median on a boxplot, ask students for the meaning of the word 'median'. Point to particular features of the displays and discuss what they are showing, asking students to recall their knowledge or explicitly introducing mathematical terminology and definition.įor example, point to one of the median lines, and ask students if they know what the line represents and the mathematical terminology for this. "How would you describe the heights of the Year 10 group?"."What do we know about the heights of the Year 7 students?".Heights of students represented in box plots Class discussionĪsk students what the two graphs show. the highest and lowest values (maximum and minimum).the first and third quartiles (Q1 and Q3).Provide two box plots for students showing The example below is from a Year 10 class on statistics where students are required to compare the heights of students in Years 7 and 10 using boxplots.
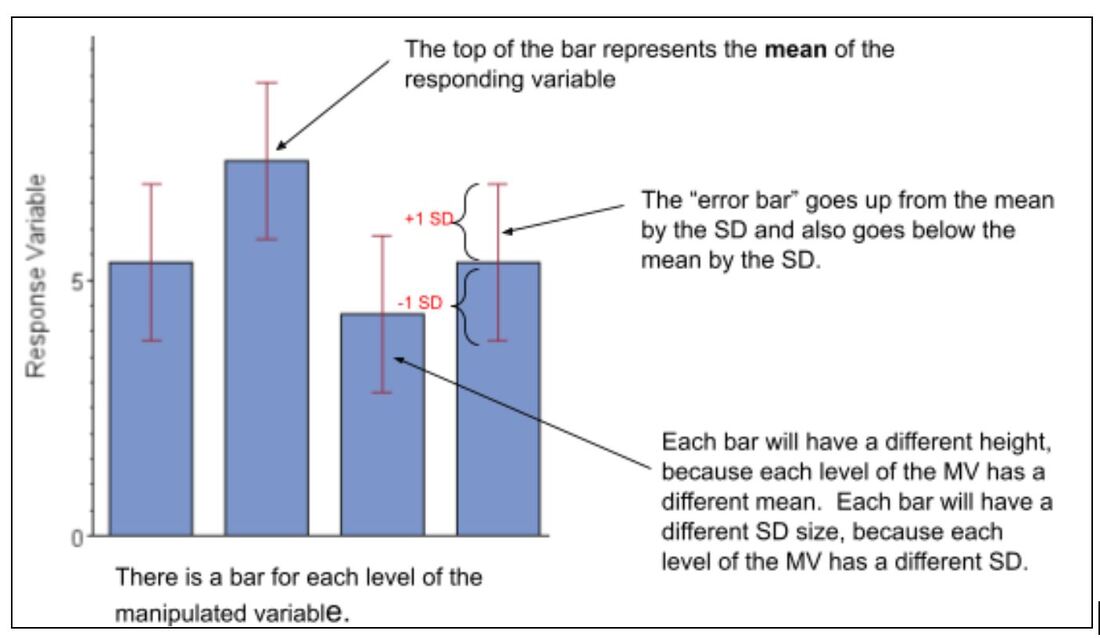
Students practise using the language that is specific to the type of graph chosen, by comparing and contrasting the features of the two displays.Įxample of using language related to box plots.Discuss how some features of a display can be labelled directly, while other features are found by calculation.If students do not know the terminology, the teacher introduces the relevant terminology and marks it on the display. Point to particular features of the displays and ask students if they know the mathematical terminology.Ask students what the two displays show.Providing two displays that compare the same attribute for two different groups.Teachers can introduce the language for given statistical displays by: Required terminologyįor example, when interpreting box plots, students will need to understand and use terminology such as: Teachers should explicitly teach key terms and features of displays. To develop the language to describe statistical displays, students will need to understand and use terminology relevant for that particular display. Introducing language for statistical displays Explicitly teaching terminology for function graphs.Introducing language for statistical displays.


 0 kommentar(er)
0 kommentar(er)
